2020 Challenges

It is all about Fluency and Flexibility

Fluency with the four operations is important but without flexibility is often simply procedural and limiting. Many students have only one way of ‘seeing’ a situation or find one solution to an open-ended situation and then say “I’ve finished.” They have not finished if they have not challenged themselves. They have not finished if there has not been some change or stretch in their thinking or in the connections that they are making.

Our 2020 challenges are designed to be open ended and adaptable through what if or what else type questions.

The Problem for Years 1 – 3

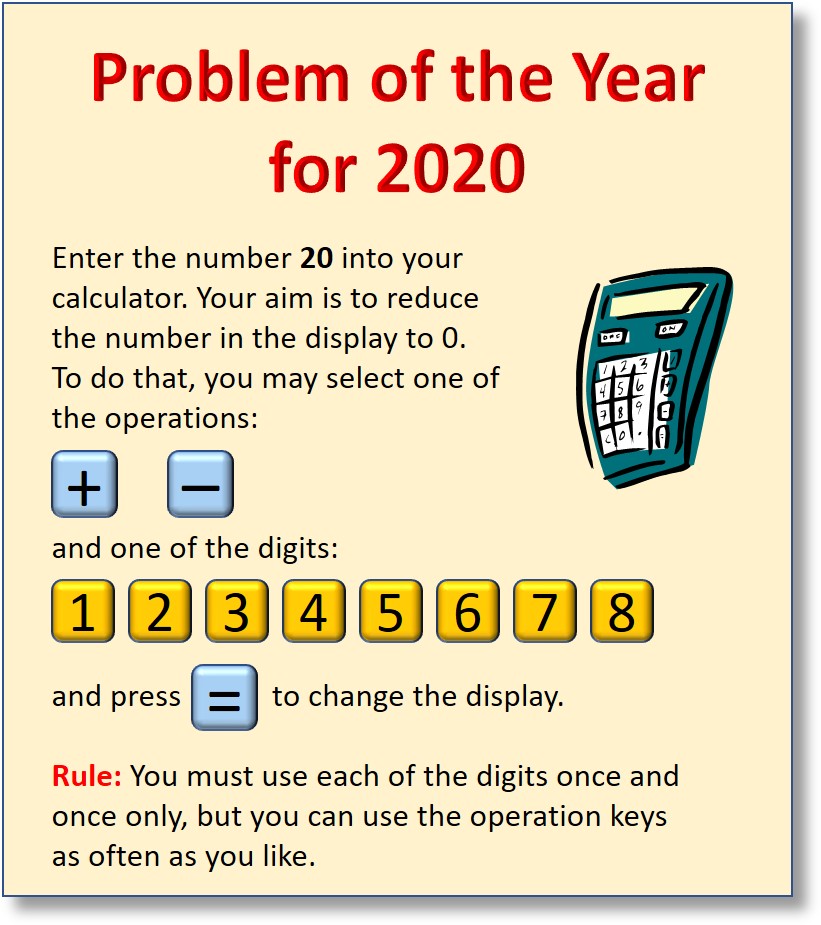
Using a calculator is not vitally important to find solutions to this problem, but it does take the stress off making additions and subtractions correctly. What does help is finding what the numbers 1 – 7 add to.

            1 + 2 + 3 + 4 + 5 + 6 + 7 = 28, which is 8 too many.

But if we add 8 to our starting number of 20 then we can subtract the numbers 1 – 7 one by one and the display will come down to 0.  It goes like this:

Step	Rationale
20 + 8 = 28	Let’s start by adding 8.
28 – 7 = 21	We can now subtract the numbers 1 – 7 …
21 – 6 = 15	step by step …
15 – 5 = 10	… by step
10 – 4 = 6	And so on.
6 – 3 = 3
3 – 2 = 1
1 – 1 = 0	Mission accomplished!

We also know that 3 + 5 = 8 and so we can add those two numbers first and then subtract all the rest:

            20 + 3 + 5 = 28, followed by

            28 – 8 – 7 – 6 – 4 – 2 – 1 = 0

There are two more ways of making 8 with two numbers, 2 + 6 = 8 and 1 + 7 = 8, and that gives use two more ways of reducing the total of 20 down to 0. What if we could find three number that add to 8! Yes, there are two possibilities here. 1 + 2 + 5 = 8 and 1 + 3 + 4 = 8; and subtracting those three numbers and adding all the rest will give us the last two possible key sequences.

Over to you, now. We’d love to hear from you or you class as to how they went with this problem.

The Problem for Years 4 – 7

Our first solution used the idea of adding a smallish number to make the display divisivble by a large number. We started with

      2020 + 5 = 2025, whose digits now add to 9 and so 2025 is divisible by 9.

Step	Rationale
2020 ÷ 5 = 2025	Let’s make the value in the display divisible by 9
2025 ÷ 9 = 225	Divide by 9 … and the result is also divisible by 9.
225 ÷ 3 = 75	3 is a factor of 9, so we know that we can divide by 3.

The only factors of 75 are numbers that we have used already, so we used + 2 to take the display to a number that was divisible by 7. And after that … it was just a matter of reducing 11 to 0 using the remaining numbers 1, 4, 6 and 8. Too easy!

Step	Rationale
75 + 2 = 77	This step gave us a way of using up 7, which is not always an easy thing to do.
77 ÷ 7 = 11	We now have 1, 4, 6 and 8 left. What should we do?
11 + 1 = 12	Make it up to 12, which leaves plenty of options and then …
12 ÷ 6 = 2
2 × 4 = 8
8 – 8 = 0	A quick check shows that all the digits 1 – 9 have been used once and once only.

We had another go, and our alternative approach was to see how close we can get to 2020 using multiplication … and maybe an addition or subtraction to adjust the running total. We started with the largest numbers first:

9 × 8 = 72

72 × 7 = 504

This looks promising. Let’s adjust with an addition

504 + 1 = 505

505 × 4 = 2020

This leaves us with 2, 3, 5 and 6 to use up and there are a few ways in which this can be done! So a very different key sequence that turns 2020 into 0 is:

Step	Rationale
2020 ÷ 4 = 505	We have to undo the multiplication that took us to 2020.
505 – 1 = 504	This step undoes the addition of 1
504 ÷ 7 = 72 72 ÷ 8 = 9 9 ÷ 9 = 1	These steps undo the multiplications by 9, 8 and 7.
1 + 6 = 7 7 + 3 = 10 10 ÷ 5 = 2 2 – 2 = 0	Here are three simple step that use the four remaining digits and bring us back to 0

Over to you, now. We’d love to hear from you or you class as to how they went with this problem.

The Sting

Yes, this is definitely adding pressure, as you have to have a +, – , × and ÷ in the sequence as well as finding a use for the decimal point.

But once you have noticed that 2025 is divisible by 9 twice, a division by 81 will make a big reduction on the size on the number in the display.

We found a sequence that works:

Step	Rationale
2020 + 5 = 2025	Let’s make that our starting point
2015 ÷ 81 = 25	Now for the decimal point.
25 × ·36 = 9	That does it!
9 – 9 = 0

Maybe your maths gurus will find another one!