The Last Number
NOTE: This went out as a newsletter to subscribers last week, and I have had SO much positive feedback that I have added it to the blog – thanks everyone! Ann
“100 is the last number there is”, one student told me. She had drawn lots of circles and counted as she went along stopping at 100. “Why did you stop?” I asked. Her answer was “Because 100 is the last number there is.” “What about 123?” I asked, to which she replied “Oh, that’s not a real number. You don’t count to 123.”
I find lots of students across all grades who cannot count beyond 100, or who cannot continue a count on pattern through the hundreds, e. g. 81, 91, 101,111 …
As usual I put my head in that all too familiar space and ask: “Why/How can this be so?”
Some of my conclusions, which I have been checking out with teachers are:
- Year 1 teachers count to 100 (not usually beyond) with their students but in Year 2 and beyond we focus on place value activities, partitioning numbers and reading and writing numbers with less focus on counting and counting patterns.
- Rote counting can be boring and laborious, (it doesn’t have to be though)
- Counting patterns are usually explored not through counting but through ‘finding the missing numbers and fill in the blanks on a worksheet type of activities, such as:

Most counting is count-on, with less attention being given to count-back. I wonder if that is why subtraction causes more difficulty than addition. In this example,
it is essential to be able to count back 3 and bridge back through the 10.
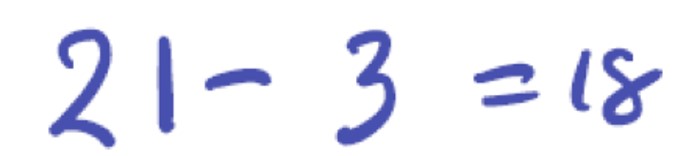
Counting is usually limited to whole numbers only. Counting in fractions and decimals is important as well.

Without counting in decimals many students will carry out ‘overflow’ counting (as in the example above) which means as in the which means students cannot bridge through the next whole number, rather they treat the decimal fraction as whole numbers counting from 0.9 to 0.105. This was actually a NAPLAN ‘continue the sequence’ question.
So, what is to be done? Counting Daily is to be done!
Schools working with me are counting with their students every day, at whatever level that means.
It is not necessary to count long sequences, but it is necessary to plan counting on and back sequences that bridge a 10, a 100, a 1000 or the next whole number.
It is also necessary to count in sequences that will make clear useful patterns and to talk about those patterns.
I find students cannot or do not stop to think about possible landing numbers, for instance as a class we may start at 87 and count on in 10s while a student crosses the room to bring back a book. Students are asked to predict what number we will land on when the walker gets back. Very rarely am I given a prediction that ends in 7.
Similarly, if we start on 3 and count on in 5s, students do not notice the pattern in the 1s position (3, 8, 3, 8, 3, 8) and act surprised when it is pointed out. Time for some what ifs. What if we started on 4, 6, 7?
Have some fun
Counting and chanting do not have to be monotonous or boring. Count in voices , e.g. robot voice, baby voice, operatic voice, rap voice, dalek voice whatever is current.
Throw out some challenges, e. g. Will it take the same amount of time to count from 100 to 15O as it does to count from 1 to 50? It took 130 giant steps to get to the library. How many small, heel-to-toe steps do you think it will take to get back? If we start at 120, 1020, … what number do you think we will land on if we count back in 1s, 10s, …?
Watch and notice
Some useful formative information comes from watching. You will hear and see:
- who counts fast and fluently (they run ahead)
- who counts fluently at an even pace
- who comes in just behind (listening and following)
- who loses count and where and whether they pick up the flow again (they need more practice)
- who doesn’t join in (they may need support or a nudge to join in).
Counting back is vital
We do much more counting on with students than we do counting back and this impacts on their disposition and strategies for subtraction.
The entry point to subtraction is fluency with the number before, 1 less than, 2 less than and then 3 less than a given number. Clearly this derives from fluent counting back.
Similarly, being able to bridge back through 10 is crucial. 11, 10, 9, 8, for 11-3 is an important step, also derived from counting back.
Observations of students’ fluency with count back 1, 2 and 3 is frequently disappointing. We talk about this and provide strategies for early subtraction in our latest package.
So …
